
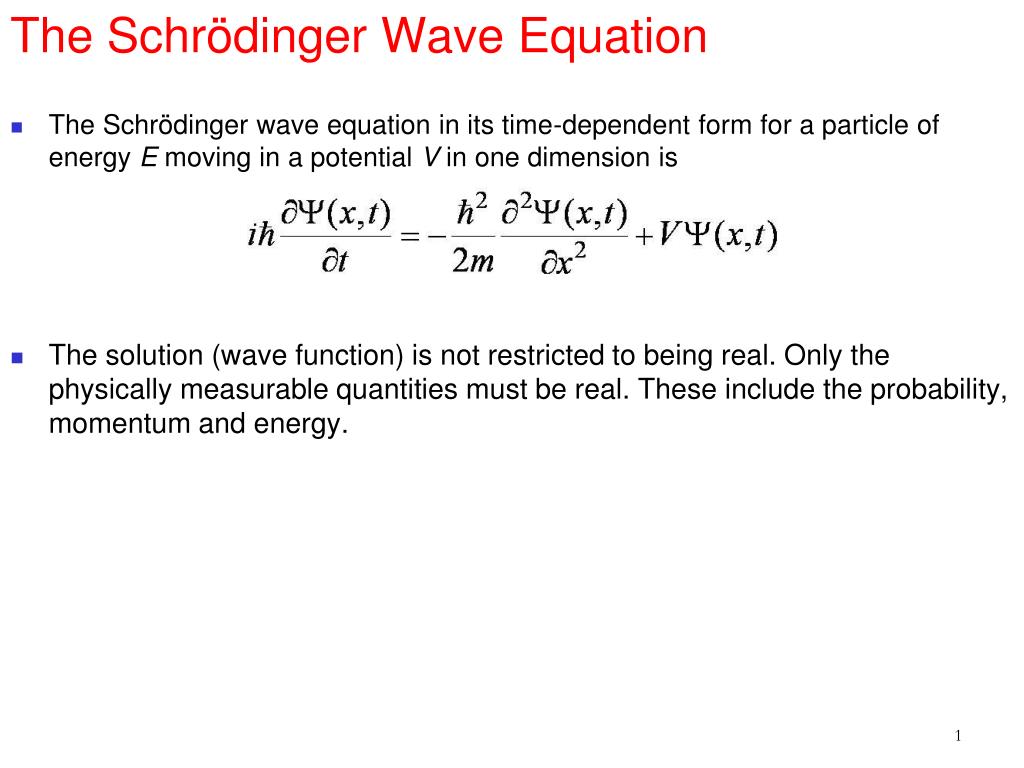
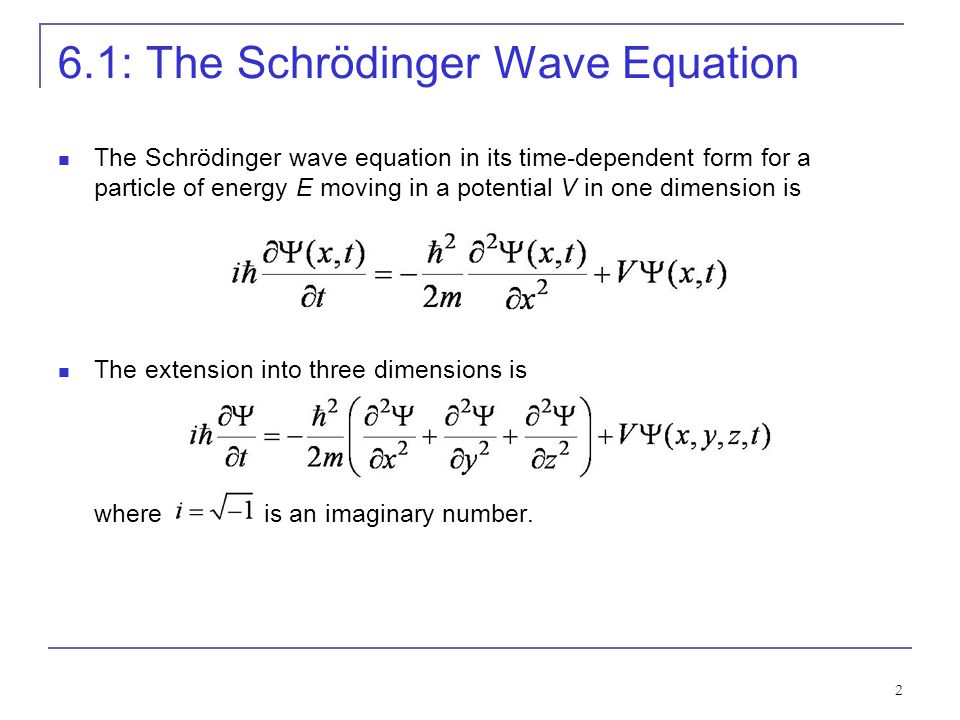
However, this choice will become clear in the next section when we discuss classical matter waves. Here, r combines the Cartesian coordinates of the particle in three space dimensions and t is the coordinate time.Īt this point, the use of a complex, rather than a real, valued wave is by no means obvious.
In the second section ( Appendix), we provide more insight into the Van Vleck determinant by considering a 1D description.Ĭonsisting of the real-valued and positive amplitude A = A( r, t) and the real-valued phase θ = θ( r, t). In the first section ( Appendix), we use standard relations of vector calculus to obtain the building blocks of our mathematical identity. To focus on the essential ideas, we have moved detailed calculations or introductory material to an appendix. Our article is organized as follows: we first establish the mathematical identity and then turn to the nonlinear wave equation corresponding to a classical matter wave the next section is dedicated to the task of arriving at the linear Schrödinger equation and we conclude by summarizing our results and providing an outlook. It is for this reason that the wave equation is nonlinear. Indeed, in the classical matter wave, this coupling is broken the phase still determines, via the continuity equation, the dynamics of the amplitude, but the equation of motion of the phase (i.e., the Hamilton–Jacobi equation) is independent of the amplitude. It is this mutual coupling between amplitude and phase that defines a quantum matter wave and ensures the linearity of the wave equation. Iii) However, a linear wave equation (i.e., the Schrödinger equation) emerges from our mathematical identity when we couple amplitude and phase in a democratic way (i.e., the phase determines the dynamics of the amplitude, and vice versa). Ii) A description ( 16 – 20) of classical statistical mechanics in terms of a classical matter wave whose phase is given by the classical action and is governed by the Hamilton–Jacobi equation ( 21), and whose amplitude is defined by the square root of the Van Vleck determinant ( 22) and satisfies a continuity equation ( 16 – 18, 22, 23), leads via our mathematical identity to a nonlinear wave equation. 2), we assume that we search for a wave equation for a scalar wave containing only a first-order derivative in time and a second-order derivative in space, and we establish a mathematical identity involving derivatives of a complex-valued field.
I) Starting from the nonlinear wave equation ( Eq. In this article, we obtain the Schrödinger equation ( Eq. There are, of course, many ways ( 8 – 15) in which to obtain the time-dependent Schrödinger equation, with the most prominent being the one developed by Feynman ( 14) based on the path integral. 2 is the fact that energy and momentum are obtained from the Hamilton–Jacobi theory by taking time and space derivatives of the action, as we discuss in the following. Here, Q is a nonlinear potential that depends on ψ (cl) ( Eq.


 0 kommentar(er)
0 kommentar(er)
